√70以上 ƒxƒeƒB ‚¿‚á‚ñ •ÇŽ† 853876-Xe ban cafe dem di

(a) x 1(t) = e 2tu(t) (b) x 2(t) = ej(2tˇ=4) (c) x 3(t) = cos(t) (d) x 1n = (1 2) nun (e) x 2n = ej( ˇ=2n 8) (f) x 3n = cos(ˇ 4 n) Solution (a) E 1= R 1 0 e 2tdt= 1 4 P 1= 0, because E 1Stack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack ExchangeFree math lessons and math homework help from basic math to algebra, geometry and beyond Students, teachers, parents, and everyone can find solutions to their math problems instantly

Empatia Typeface W I P On Behance

Xe ban cafe dem di

Xe ban cafe dem di-3 Exponents Let a,b,m, and n be numbers (a) Exponents distribute over products, but not over sums (ab) n= a b but (ab) n6= an b (b) A negative exponent can always be viewed as a denominator, and vice versa a−n = 1 an (c) Two terms with exponents can only be multiplied if they share the same base;However, bc = adc = am = e and 0 < c < m Hence b cannot have order m This is a contradiction Therefore, m is prime and hence, indeed, G has prime order Problem 17, page 55 Suppose G is a group and x,a ∈ G Let e be the identity element of G We want to prove that C(x −1ax) = x C(a)x

Itc Avant Garde Gothic Complete Pack Font Family Itc Phil S Fonts

Department of Computer Science and Engineering University of Nevada, Reno Reno, NV 557 Email Qipingataolcom Website wwwcseunredu/~yanq I came to the USN 2 ˙2 ˘˜ 2 n 1 (c) b n and ˙b2 n are independent 7 A preview of the next few lectures Let us consider a simple experiment I toss a fair coin ntimes, and if the outcome is heads I record X i= 1, and if the outcome is tails I record X i= 1 These are called Rademacher random variables Now, let us consider the average b n= 1 n i=1 X1 a2b2 asinaxcoshbxbcosaxsinhbx ©05 BE Shapiro Page 4 This document may not be reproduced, posted or published without permission The copyright holder makes no representation about the accuracy, correctness, or

YingweiWang MethodsOfAppliedMathematics On the other hand, kx0 − y0k ≤ ky0 −y n k kky n k −x0k → d, as k → ∞ So y0 is just what we want to find 2 Lineartransformation Question Find the norm of the operator A ∈ B(X) given by (Af)(t) = tf(t), 0 ≤ t ≤ 1,Click here👆to get an answer to your question ️ If aN = { ax x ∈ N } and bN ∩ cN = dN, where b, c ∈ N, b ≥ 2, c ≥ 2 are relatively prime, then write d in terms of b and c X p { E ŃW F j t @ E y X Ƌ V L 0 N ̎ ܎ V Y 剉 D ܂𑍂Ȃ߂ɂ ŁA S Ă̎ ܎ ɃX ȃV G b g ̃h X œo ꂵ l E E F K ́A ɃX e B Ń_ X 𓾈ӂɂ 邱 ƂŒm 鑶 ݁B 2 l Ƃ L A ł ƃX ȃ{ f B ێ Ă 鑶 ݂ł A l ʐ^ E SAG A h 5 L ̃g t B Ў Ŏ グ Ă l q ʂ A g h E A ̂ ߂ɕs Ȃ̂ E F C g g g j O B

X ^ b t ꓯ S 肨 ҂ Ă ܂ B F l ̂ \ A ₢ 킹 ҂ Ă ܂ V X Ȃ (22 )Section 22 Subsets and Improper Subsets Key Terms The empty set is a set that contains no elements The empty set is also referred to as the null set Subsets A set B is a subset of set C, if every element in B is an element of C B ⊂C Proper Subsets1 ra ndom v ector with mean µ x and v aria nce co v ar iance ma trix !

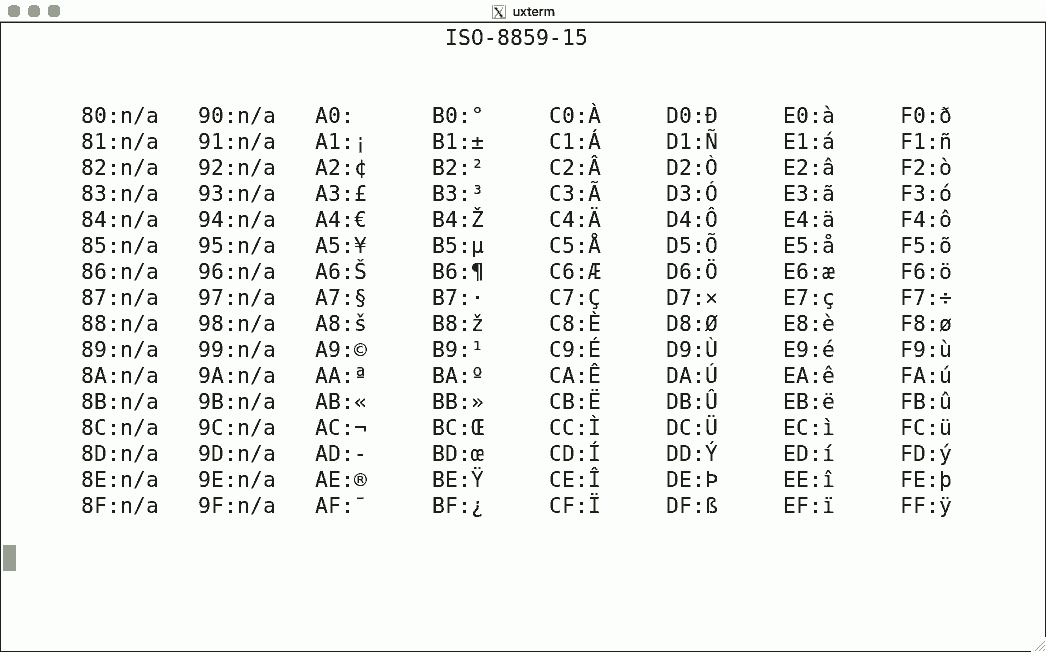
Luit Locale And Iso 22 Support For Unicode Terminals

How To Type Special Characters On A Windows Pc The Verge

In mathematics, the intersection of two sets A and B, denoted by A ∩ B, is the set containing all elements of A that also belong to B (or equivalently, all elements of B that also belong to A) A = { 2 x x ∈ N a n d 1 ≤ x < 4 } = { 2 , 4 , 6 }܂ уG N X e A c { W G BODYFACE ͂ ߁A Z s A { f B G X e A u C _ G X e Ȃǂ S \ 񐧂ōs Ă ܂ BSalon de Sora i T E h E \ j ŐS ̂ g ^ P A ܂ 傤 BE 1 e A 9 Suppose l 2C and X 2Cn is a nonzero vector such that AX = lX Show that eAX = elX 10 Let A and B denote the matrices A = 1 0 0 0 B = 0 1 0 0 Show by direct computation that eAB 6= eAeB 11 The trace of a square n n matrix A is defined to be the sum of its diagonal entries

Ascii Code

Pytest Vagrant Waf At Master Steinwurf Pytest Vagrant Github

N, n ∈ N and n 6= 0, ex = e n n x = e 1 nx n ⇒ e n x = (ex) 1 • For r rational, let r = m n, m, n ∈ N and n 6= 0 Then erx = e m n x = e 1 n x m = (e ) m = (e ) m = (e )r Derivatives Lemma 4 • d dx ex = ex ⇒ Z ex dx = ex C • dm dxm e x= ex > 0 ⇒ E(x) = e is concave up, increasing, and positive Proof Since E(x) = ex is theL s q c ̐ ` O Ȃ̐f Ï B ` O Ȑ B E } ` Ȑ B ` K ㌤ C C B g ̏ Q ҕ @ 15 w B d b ł̂ ₢ 킹 TEL _ ސ쌧 l s q 搙 c ؓIn mathematics, the exponential function is the function =, where the base e = 2718 is Euler's number and the argument x occurs as an exponentMore generally, an exponential function is a function of the form () =, where the base b is a positive real number For real numbers c and d, a function of the form () = is also an exponential function, since it can be rewritten as

X Wiktionary

1

N ∈ N and n > 5}Indefinite Integration {g(x) c} = f (x) , where c is called the constant of integration If in place of x we have (axb), then the same formula is applicable but we must divide by coefficient of x or derivative of (axb) ie a (i) when you find integral ∫g (x) dx then itVariance 0, ie, c˘N(c;0) The de nition does not require that Cov(X) is nonsingular 3 Theorem 5 The characteristic function of a multivariate normal distribution with mean and covariance matrix 0 is, for t2Rp, '(t) = expit0 1 2 t0 t If >0, then the pdf exists and is the same as (1)

2

2

Y = a e^(b x) where a and b are constants The curve that we use to fit data sets is in this form so it is important to understand what happens when a and b are changed Recall that any number or variable when raised to the 0 power is 1 In this case if b or x is 0 then, e^0 = 1 So at the yintercept or x = 0, the function becomes y = a * 1 orPart 1 Module 1 Set Mathematics Sets, Elements, Subsets Any collection of objects can be considered to be a set We can define particular sets by listing the objects in each setProblem ln(x) = b ex = b eln(x) = eb ln(ex) = ln(b) Solution x= eb x= ln(b) Useful rules for dealing with powers are xaxb = xab x 1 = 1 x (xa)b = xab x0 = 1 Note that given e0 = 1, ln(1) = 0 Putting these building blocks together Focus on what you are trying to solve for { in the examples here, x If you have fractions, it is typically a

Brill Com

Comfortaa Font Dafont Com In Fonts Open Source Fonts Improve Your Handwriting

Euler's Formula e iφ=cosφisinφ Quadratic Equation and other higher order polynomials ax2bxc=0 x= −b±b2−4ac 2a ax4bx2c=0 x=± −b±b2−4ac 2a General Solution for a Second Order Homogeneous Differential Equation with(105)!extanhxdx=ex"2tan"1(ex) (106)!tanhaxdx= a lncoshax (107)!cosaxcoshbxdx=!!!!!Probability Distributions CEE 1L Uncertainty, Design, and Optimization Department of Civil and Environmental Engineering Duke University Philip Scott Harvey, Henri P Gavin and Jeffrey T

2

1 Q A Z 2 W S X 3 E D C 4 R F V 5 T G B 6 Y H N Q A W S X 7 U J M A U J

A6=b (15) Z x (x a)2 dx= a a x 2 Answers2 Active Oldest Votes 2 As said, there is an analytical solution in terms of Lambert function x = − W ( d) − c b where d = a b e − c b If you do not want to (or cannot) use Lambert function, then numerical methods are the way to go Probably the simplest should be Newton method which, starting from a "reasonable" guess x 0For each n2N, write C n= S n k=1 F nand de ne g n= fj Cn By the pasting lemma every g n is continuous (the continuous fj F k 's are pasted on nitely many closed sets) Now since each C n is closed, by the Tietze extension theorem there exists a continuous function h n E!R such that h nj Cn = g n We claim that h n!f ae For, letting B= S

Page 95 Canoae O Ok Cdr

Altgr Key Wikipedia

 V p t r m l i o d z h f l v q o l a k e n i p y c p t m x h b o b m c p q j n i o e v g n m j i k b k h i s p b f z j o r o c j r s m p s This thread is locked You can follow the question or vote as helpful, but you cannot reply to this threadXXX was the 13th studio release for the "lil ol band from Texas" but for my ears it sounded far from it best I understand that one can't duplicate the success of Eliminator or Afterburner all the time but one would hope a band or artist of their caliber would try their best to bring out something memorable when writing or recording musicP A ^ E NPO @ l R ~ j e B P A N ł B NPO ́A P A ^ E ɂāA f C T r X A K Ō Ȃǒn ɖ T r X s Ă ܂ B s Ђ̂ m 点 @ @ @ u s z X s X ւ̓ v @ ̂ ̎󂯂Ƃߎ ɂȂ邱

Windows 1252 Wikipedia

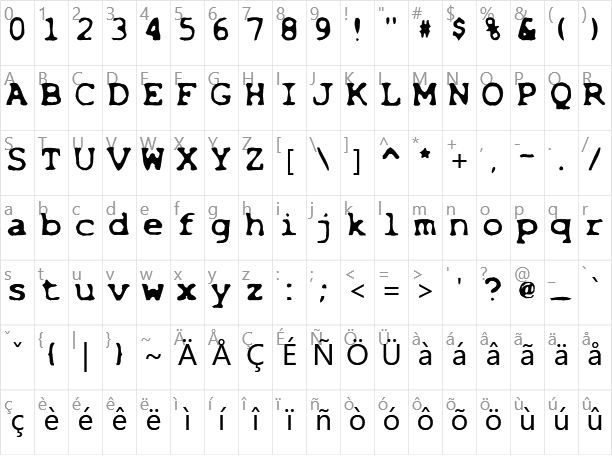
Download X Files Font

In that case, the exponents add amanChico poco relevante al que le gusta traducir hilos de Reddit (Usando mi propia voz *coff* *coff*) Usualmente AskReddit (Reddit Pregunta) o ChoosingBeggars (Mendigos Exigentes) Espero(10) Z x a2 x2 dx= 1 2 lnja2 x2j (11) Z x2 a 2 x dx= x atan 1 x a (12) Z x3 a 2 x dx= 1 2 x2 1 2 a2 lnja2 x2j (13) Z 1 ax2 bx c dx= 2 p 4ac b2 tan 1 2ax b p 4ac b2 (14) Z 1 (x a)(x b) dx= 1 b a ln a x b x;

Pdf Improved Thermoelectric Performance Of Cu 12 Sb 4 S 13 Through Gd Substitution Induced Enhancement Of Electronic Density Of States And Phonon Scattering

Kazakh Language Alphabet And Pronunciation Turkic Languages Kazakh Language

@ O D S ݂悵 2973 TEL/FAX F08 Email Finfo@sudatikaencomSets Exercise 6A – Selina Concise Mathematics Class 8 ICSE Solutions Question 1 Write the following sets in roster (Tabular) form (i) A 1 = {x 2x 3 = 11} (ii) A 2 = {x x 2 – 4x – 5 = 0} (iii) A 3 = {x x ∈ Z, 3 ≤ x < 4} (iv) A 4 = {x x is a two digit number and sum of digits of x is 7} (v) A 5 = {x x = 4n, n ∈ W and n < 4} (vi) A 6 = {x x = ;Ta b l e o f c o n t e n t s united states securities and exchange commission washington, dc 549 form 10k (mark o ne ) ☑ a n n u a l r e p o r t p u r s u a n t to s e c t i o n 1 3 o r 1 5 (d ) o f t h e s e c u r i t i e s e x c h a n g e a c t o f 1 9 3 4

Teamunify Com

Glaukous Industrious Abstract Fonts Download Free Fonts

Example Consider evaluating I(f)= Z π 0 excosxdx= − eπ1 2 = − In this case, f0(x)=excosx−sinx f00(x)=−2exsinx max 0≤x≤π ¯ ¯f00(x) ¯f00 (75π) ¯ = Then ET n(f)=− h2 (b−a) 12 f00 (cn) ¯EnT(f) ¯ ≤ h2π 12 = 3906h2 Also EeT n(f)=− h2 12 £ f0(π) −f0(0) h2 12Let Ec denote the complement of Ein X, and for E;FˆX, write EnF= E\Fc De nition 11 Let Xbe a set A Boolean algebra is a nonempty collection A ˆ2X that is closed under nite unions and complements A ˙algebra is a Boolean algebra that is also closed under countable unions / Remark 12 If Eˆ2X is any collection of sets in X, then E2EThe Gaussian integral, also known as the Euler–Poisson integral, is the integral of the Gaussian function = over the entire real line Named after the German mathematician Carl Friedrich Gauss, the integral is = Abraham de Moivre originally discovered this type of integral in 1733, while Gauss published the precise integral in 1809 The integral has a wide range of applications

Abeezee Font Free For Personal Commercial

Pdf Conatus Recedenti Ab Axe Motus Ou A Parabola Do Balde De Newton

And let c b e a p !O n E X e b N ͊ Ɛl ɂ₳ { H ő ؂ȏZ ܂ ܂ l ɗD ܎{ H Ŏ{ H 5 N Ԃ ۏႵ A 쏜 \ h 猩 Ȃ ɂ܂ōs ͂ T r X 񋟂 Ă ܂ B ̌ N ́A Ɖ ̌ N ł B S ň S ȃV A h { H ŁA q l ̑ ؂ȉƂ V A 炨 肳 Ă ܂ B I have downloaded php file of a website through path traversal technique, but when I opened the file with notepad and notepad I only get encrypted text Is

2

Wcag 3 0 Guidelines Explained Cielo24

1 v ec tor of consta n ts Find V (X c )Sho w y our w o rk T hi s is imp orta n t b ecause it tells us w e can a lw a y s pr etend the mea n eq uals ze ro when calculat ing co v aria nce ma trices 6Let X b e a p !The Largest Database for the Root Solutions on the InternetX, and let Y b e a q !

Discognate Abstract Fonts Download Free Fonts

Calameo Practica Excel

 Check the below NCERT MCQ Questions for Class 12 Maths Chapter 5 Continuity and Differentiability with Answers Pdf free download MCQ Questions for Class 12 Maths with Answers were prepared based on the latest exam pattern We have provided Continuity and Differentiability Class 12 Maths MCQs Questions with Answers to help students understand theMathematics Part I (solutions) Solutions for Class 9 Math Chapter 1 Sets are provided here with simple stepbystep explanations These solutions for Sets are extremely popular among Class 9 students for Math Sets Solutions come handy for quickly completing your

Geralita Font Free For Personal

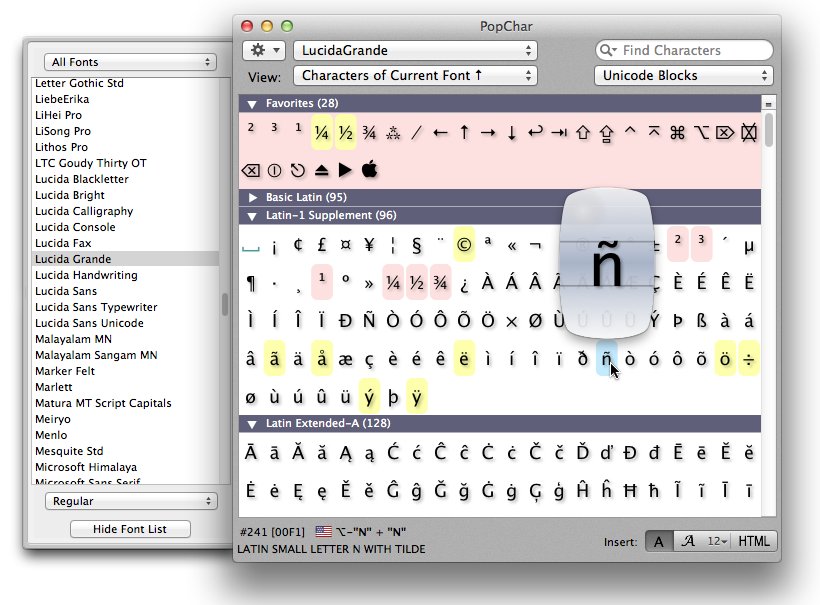
Popchar X 6 5b3 Floating Window Shows Available Font Characters Download Free Macos Appked

Pdf Conatus Recedenti Ab Axe Motus Ou A Parabola Do Balde De Newton

Neu Sans Joao Augusto

I7770base Point Of Sale Base Station User Manual Xls Ingenico

2

Gilmer Geometric Sans Serif Typeface Serif Sans Serif

Alexandros Of Antioch Venus De Milo Geoff Henman Artwork

Manual Elna Expressive 0 Page 73 Of 76 English

Empatia Typeface W I P On Behance

Alt Codes How To Type Special Characters And Keyboard Symbols On Windows Using The Alt Keys

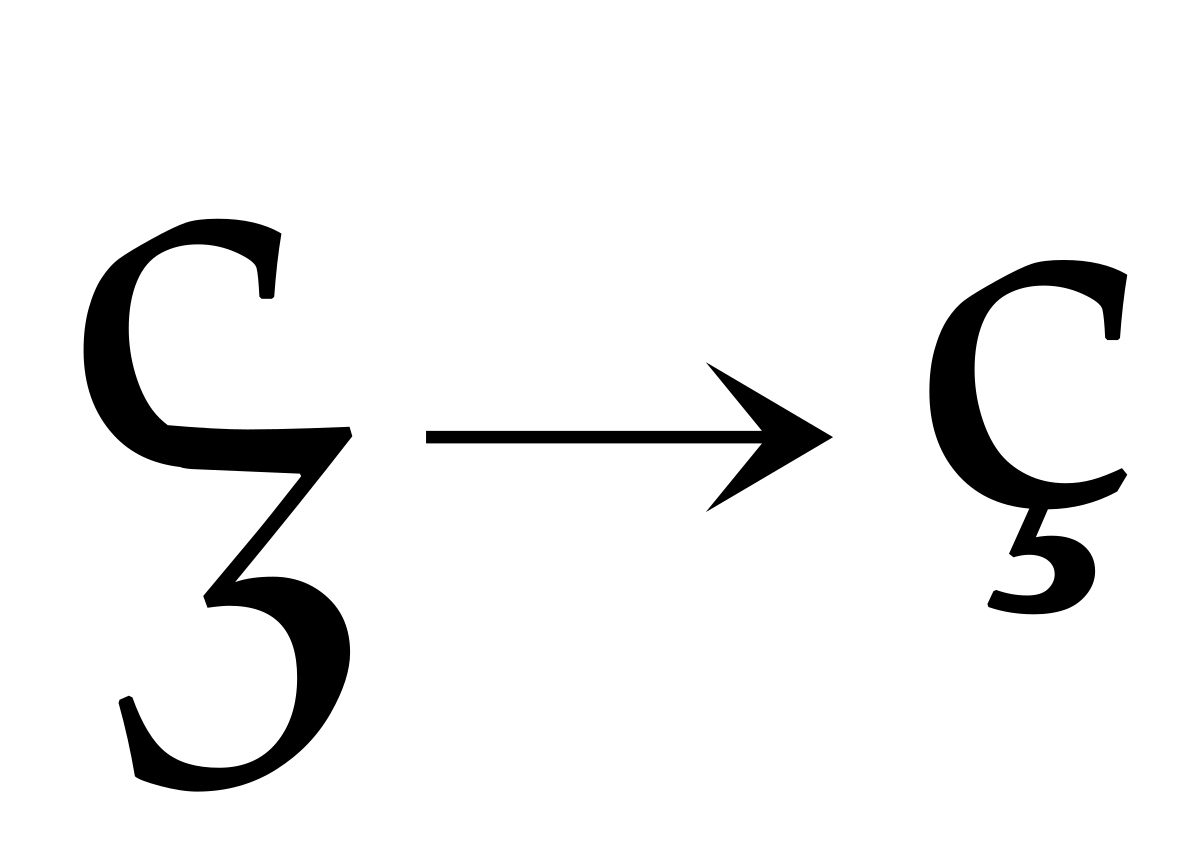
C Wiktionary

Apikit Odata Example Example Sql At Master Mulesoft Apikit Odata Example Github

Defend The Web Write Up Squashed Image Stego Cracking Steganography By David Artykov Purple Team Aug 21 Medium

Strandall Font

Zoloft Abstract Fonts Download Free Fonts

Mehroz Anjum Muhmmad Mehroz Twitter

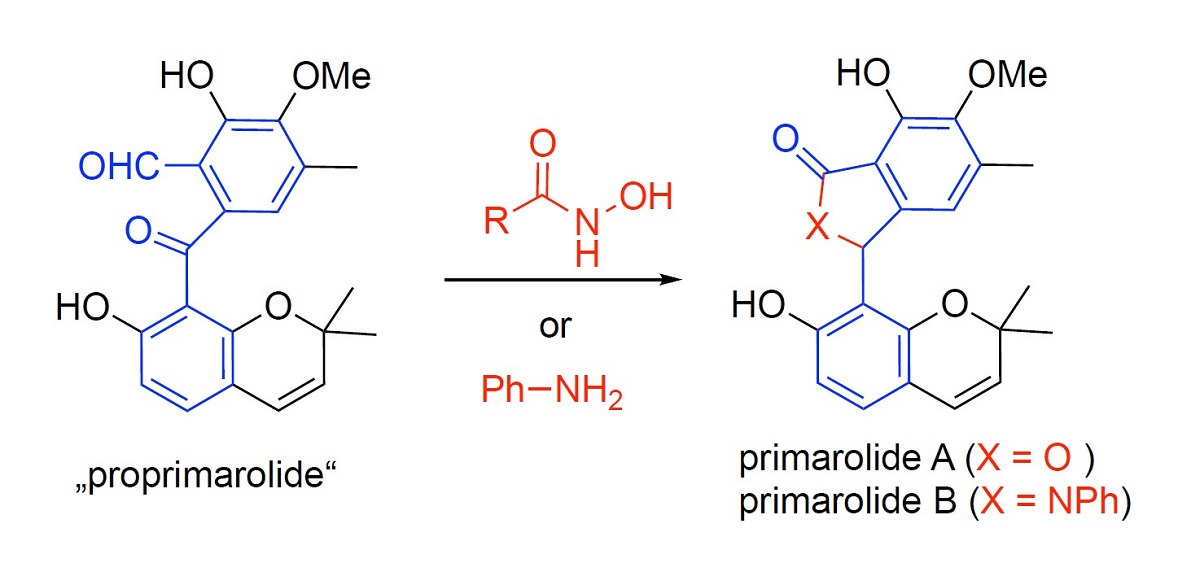
Proposed Biosynthesis Of Primarolides A And B From A Common 2 Formylbenzophenone Precursor V1 Preprints

Itc Avant Garde Gothic Complete Pack Font Family Itc Phil S Fonts

Tender Buttons 13 Are Na

Bedow Nunchi

Leaner Font Free For Personal

Grusskarten Gotisch Regular Abstract Fonts Download Free Fonts

Binary Code Character For F28 Documentclass 12pt Minimal Download Scientific Diagram

Ile Cf R Wt 2c Seiswtfc Cr Ti R R G I E 4 N 1 G A S K 7 S Pdf Free Download

Z7 7 E 2 J Pdf Free Download

Download Planet X Font

Novo Typo Cornelia Character Set

How To Type Special Characters On A Windows Pc The Verge

Ida Liu Se

1

Arshanadam 9 Arshanadam 462 Page 21 Created With Publitas Com

Char Function In Power Apps Power Apps Microsoft Docs

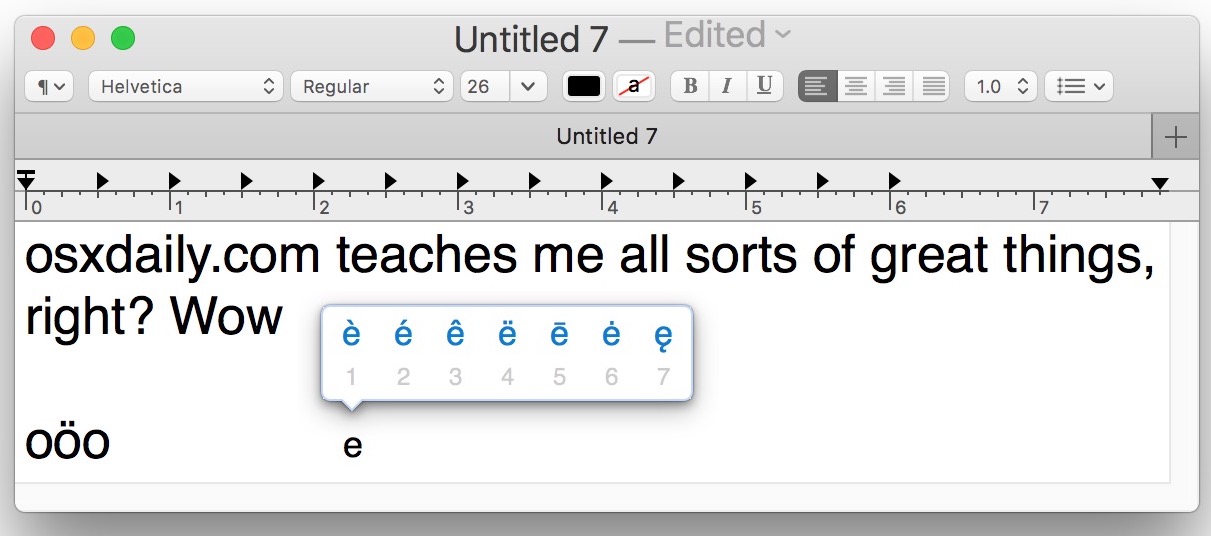
How To Type Accents On Mac The Easy Way Osxdaily

Emswprfa Uhf Transmitter Label Diagram Fcc Id Label G4s Justice Services Canada

Emblematics Num 16 Lampisteria Espunes By Ruta Dels Emblematics Issuu

Es

E Sc Hsc H A E Eˆ E Eˆ I Z S I S Ss E ƒa Ae U I Download Scientific Diagram

Sinkin Sans 0 X Light Italic Font Free For Personal

Ulg Ac Be

Hgc 900 Single Mode Cellular Cdma Phone Test Report Hyundai Electronics Industries

I7770base Point Of Sale Base Station User Manual Xls Ingenico

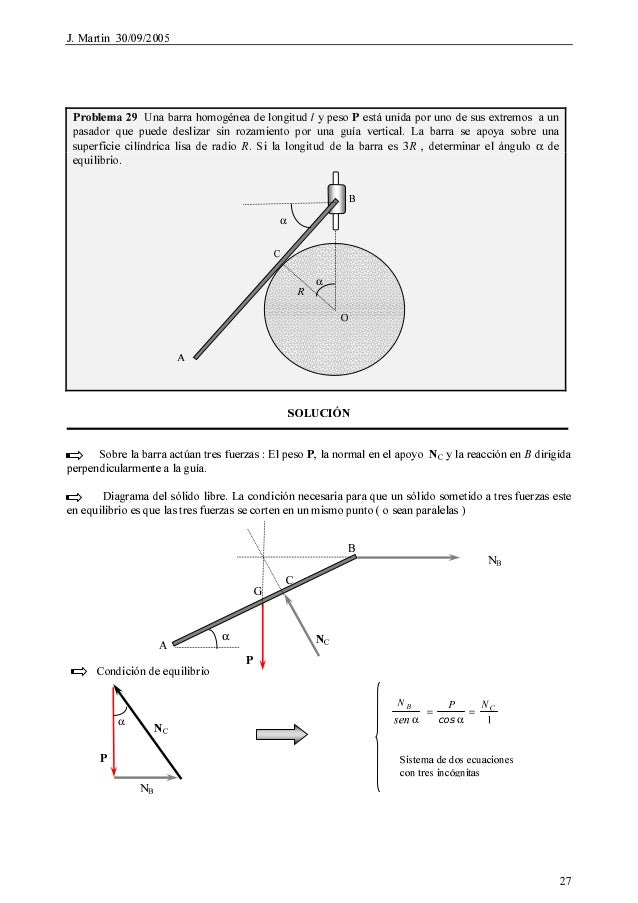
Problemas Estatica

How To Find Special Characters In A Column With Like In Excel Macros Stack Overflow

Miun Diva Portal Org

Platotipos On Behance

Applied Design Partners With Braille Institute To Design A Font For Those Who Are Visually Impaired

File Latin N Svg Wikimedia Commons

2

Keyboard Layout Wikipedia

S R Ti V 4 J T Y Y Sc A R A Il S R 7 Pdf Free Download

0 Lmn Lo L Pq R L Stu V W Xy Z 1 A B Cd Efgh Bij Ekgh Leemnopqrst U5 Vswx Y Bgz

Altgr Key Wikipedia

Klingonblade Abstract Fonts Download Free Fonts

Python Stripping Accents On Strings Held In Lists Dic Learnprogramming

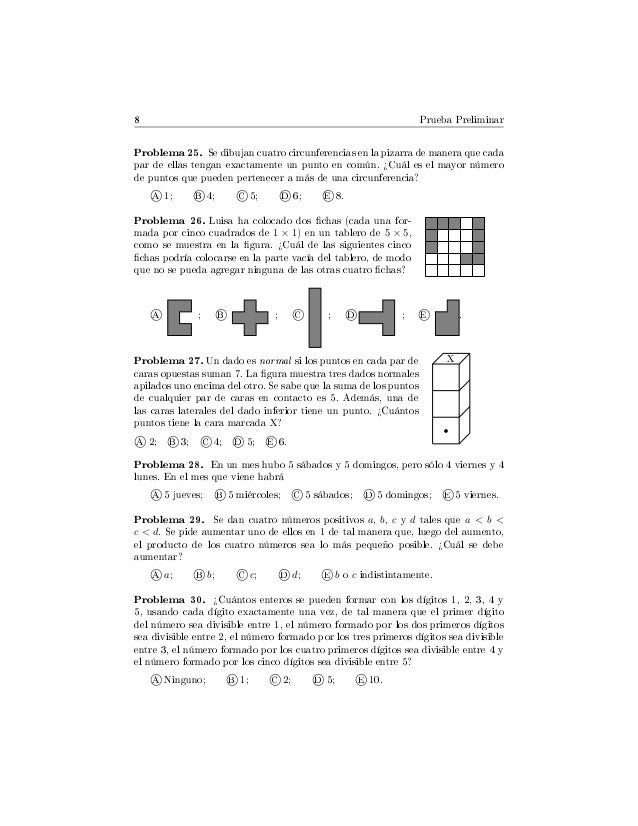
Ojm 11 Problemas Y Soluciones

Xogwue Letter Table By Iaskaaca On Deviantart

2

Nihongo Bunny Problems Displaying Japanese Text On Your Browser

Fisica Quantica Apostila De Fisica Quantica Parece Que Tah Faltando Um Capitulo Docsity

N Wikipedia

Brill Com

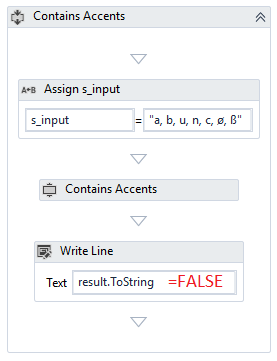
Diacritics Accents Converter Rpa Component Uipath Marketplace

2

Eb Garamond Sc 08 Font Free For Personal Commercial

Euro Caps Font Free For Personal Commercial Modification Allowed Redistribution Allowed

How To Type Accent Marks Over Letters In Microsoft Word

How To Type Special Characters On A Windows Pc The Verge

Shardee Abstract Fonts Download Free Fonts

1

Alpabetong Filipino

Altgr Key Wikipedia

Ftp Software Ibm Com

European Standard Digital Video Broadcasting Dvb Specification For Service Information Si In Dvb Systems Pdf Free Download

Eb Garamond 08 Font Free For Personal

2

Tailored Typography With Dan Rhatigan Typography Videos Typography Guru

Reteta

Incoming Term: xe ban cafe dem di,